Table 4-1. Calculating Half-wave Rectifier and Full-wave Rectifier

______________________________________________________________ Solid State Power Supplies

1

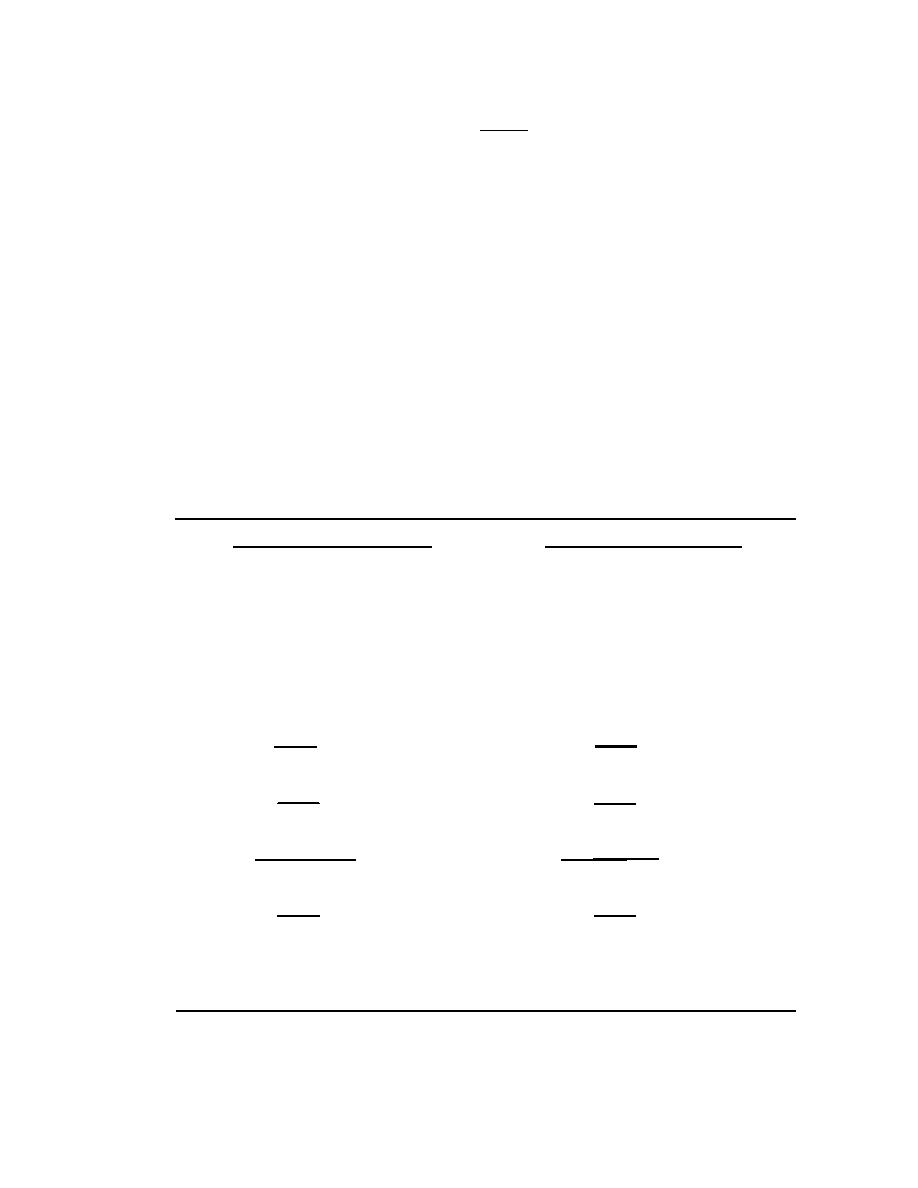
XC =

2πfC

4-48. Next, you must establish some values for the circuit. As you can see from the

calculations in Table 4-1, by doubling the frequency of the rectifier, you reduce the

impedance of the capacitor by one-half. This allows the AC component to pass through the

capacitor more easily. As a result, a full-wave rectifier output is much easier to filter than

that of a half-wave rectifier. Remember, the smaller the XC of the filter capacitor, in respect

to the load resistance, the better the filtering action. If load resistance is made small, the

load current increases, and the average value of output voltage (Eavg) decreases. The RC

discharge time constant is a direct function of the value of the load resistance. Therefore,

the rate of capacitor voltage discharge is a direct function of the current through the load.

The greater the load current, the more rapid the discharge of the capacitor, and the lower

the average value of output voltage. For this reason, the simple, capacitive filter is seldom

used with rectifier circuits that must supply a relatively large load current. Using the simple

capacitive filter in conjunction with a full-wave or bridge rectifier provides improved

filtering because the increased ripple frequency decreases the capacitive reactance of the

filter capacitor.

Table 4-1. Calculating Half-wave Rectifier and Full-wave Rectifier

FULL-WAVE RECTIFIER

HALF-WAVE RECTIFIER

FREQUENCY AT RECTIFIER

OUTPUT: 60 Hz

OUTPUT: 120 Hz

VALUE OF FILTER CAPACITOR:

30F

LOAD RESISTANCE (RL): 10κΩ

1

XC =

2πfC

.159

XC =

fC

.159

XC =

60 x .000030

120 x .000030

.159

XC =

.0018

.036

44.16Ω

88.3Ω

XC =

23 June 2005

TC 9-62

4-17