Figure 3-21. Two equal sides
(1) An equilateral triangle is also an equiangular triangle.
(2) The bisector of the angle opposite the unequal side of an isosceles triangle is the
perpendicular bisector of the base line.
(3) The perpendicular bisector of the unequal side of an isosceles triangle bisects the
opposite angle.
d. Theorem 3. If one side of a triangle is longer than another side (short side), the angle
opposite the long side is greater than the angle opposite the short side. This relationship is shown in
Figure 3-20.
3-12. Theorems for Triangle Bisectors, Altitudes, and Medians. The following theorems are for the
relationships of the triangle bisectors, altitudes, and medians.
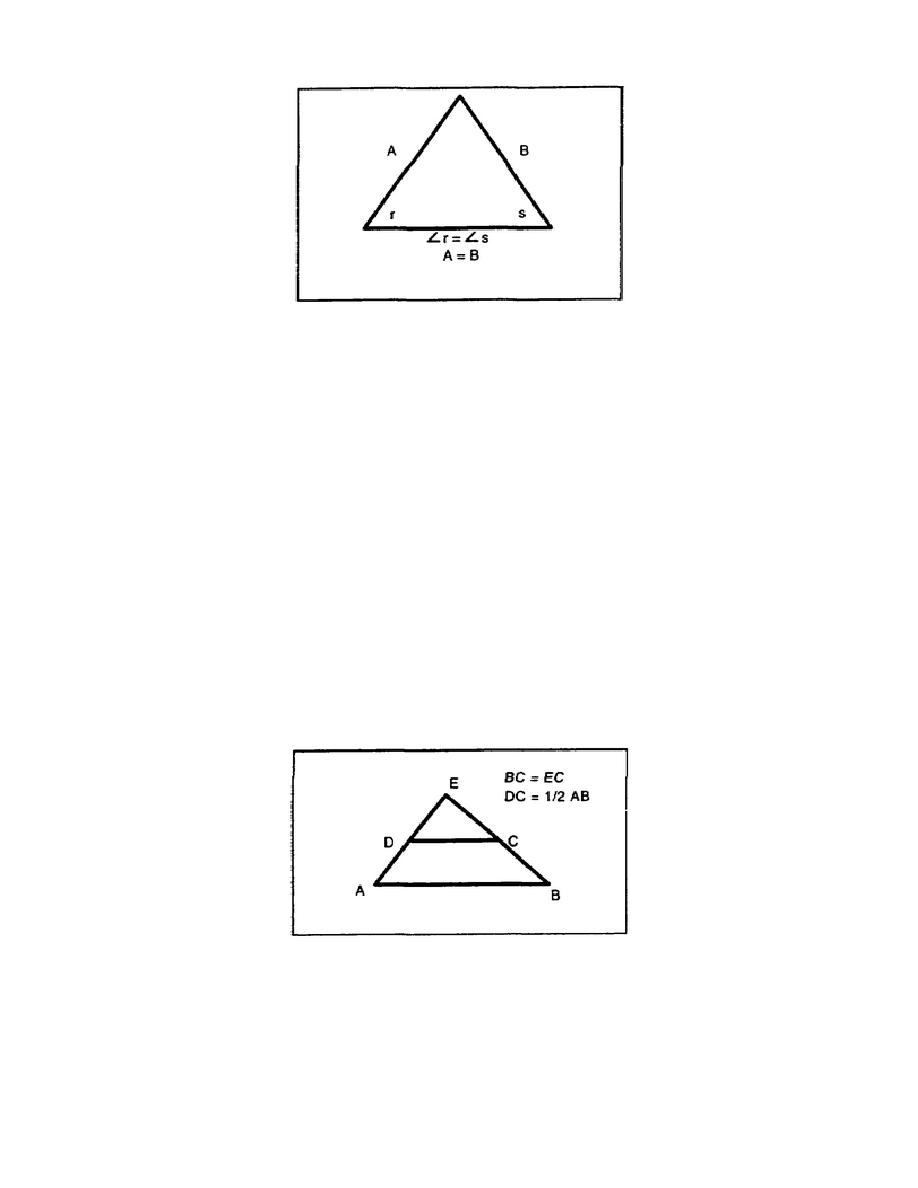
a. Theorem 1. If a line is parallel to one side of a triangle and bisects the other two sides, it is
half as long as the side to which it is parallel. In Figure 3-22, line DC is parallel to AB, line EC is equal
to BC, line ED is equal to AD, and line DC is one-half the length of AB.
Figure 3-22. Line parallel to one side and bisecting the other two sides
b. Theorem 2. Perpendicular bisectors of the sides of a triangle meet in a point that is
equidistant from the three vertices of the triangle. In Figure 3-23, page 3-14, point P is where the
perpendicular bisectors meet.
3-13
EN0591


 Previous Page
Previous Page
