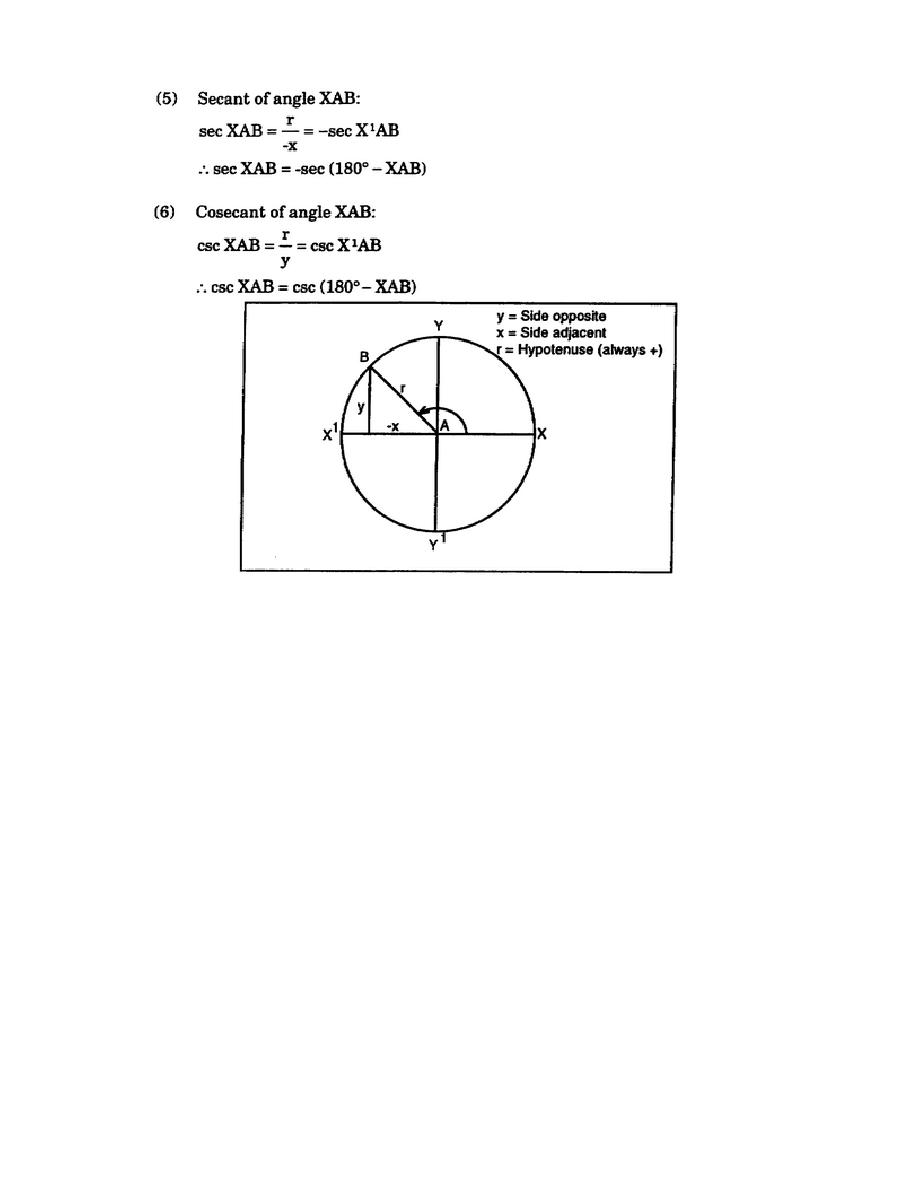
Figure 4-13. Angles between 90 and 180
h. The functions of any angle between 180€ and 270 can be expressed as the function of the
acute angle, which is found by subtracting 180 from the given angle. Assuming that angle XAB in
Figure 4-14 can have any value from 180 to 270, you can derive the following formulas for the
functions of any angle in the third quadrant.
(1) Sin of angle XAB:
sin XAB = - sin X1AB =-sin (XAB - 180)
(2) Cos of angle XAB:
cos XAB = -cos X1AB = -cos (XAB - 180)
(3) Tan of angle XAB:
tan XAB = tan X1AB = tan (XAB - 180)
(4) Cot of angle XAB:
cot XAB = cot X1AB = cot (XAB - 180)
(5) Sec of angle XAB:
sec XAB = -sec X1AB = - sec (XAB - 180€)
EN0591
4-20
Previous Page
