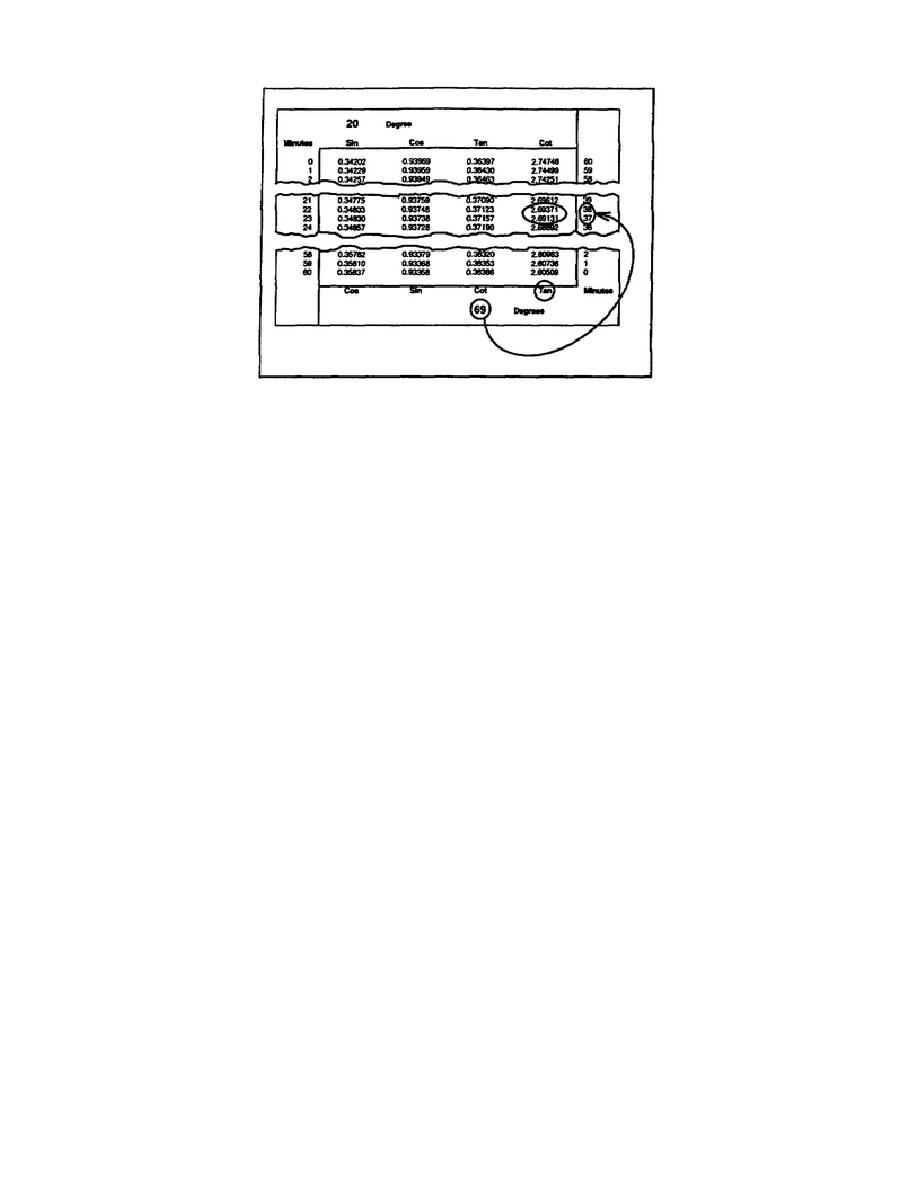
Figure 4-9. Tangents and cotangents
4-6. Functions of Obtuse Angles. Previously, you studied the functions of the acute angles of a right
triangle. As a surveyor in the field, you are often required to turn oblique angles, that is angles greater
than 90. It is necessary for you to know the functions of angles greater than 90 in order to solve an
oblique triangle. If one of the angles of a triangle exceeds 90, you no longer have a right triangle. It is
possible to express the functions of angles greater than 90 as ratios of the sides of right triangles, and
relations can be derived between such functions and the functions of acute angles.
a. To determine the functions of angles greater than 90, you will construct a pair of straight
lines intersecting at right triangles, as shown in Figure 4-10. The intersection point of the two lines is
called the origin and is labeled A. The horizontal line is labeled XX1, and the perpendicular line is
labeled YYl. Using these lines as axes of a rectangular coordinate system, a point (such as B) can be
negative when B is to the left of YY1. The y coordinate is positive when B is above XX1 and negative
when B is below XX1. Using the origin as center and any convenient radius, you can construct a circle
that will enable you to determine the functions of angles from 0 through 360. This is shown in Figure
4-11.
b. Referring to Figure 4-11, you see that the circle is divided into four equal parts, each
containing 90 of arc. These parts are called quadrants and are labeled counterclockwise from the line
AX as follows:
The first quadrant is contained in the segment of the circle XAY and is lettered with the
Roman numeral I. It contains the angles between 0 and 90.
The second quadrant is contained in the segment of the circle YAXP and is labeled II. It
contains the angles between 90 and 180.
EN0591
4-16


 Previous Page
Previous Page
