a2 = b2 + c2 - 2 bc cos A
b2 = a2 + c2 - 2 ac cos B
c2 = b2 + a2- 2 ab cos C
If the given included angle is obtuse, remember that its cosine is negative and this sign must be applied
when the value of the cosine is substituted in the formula.
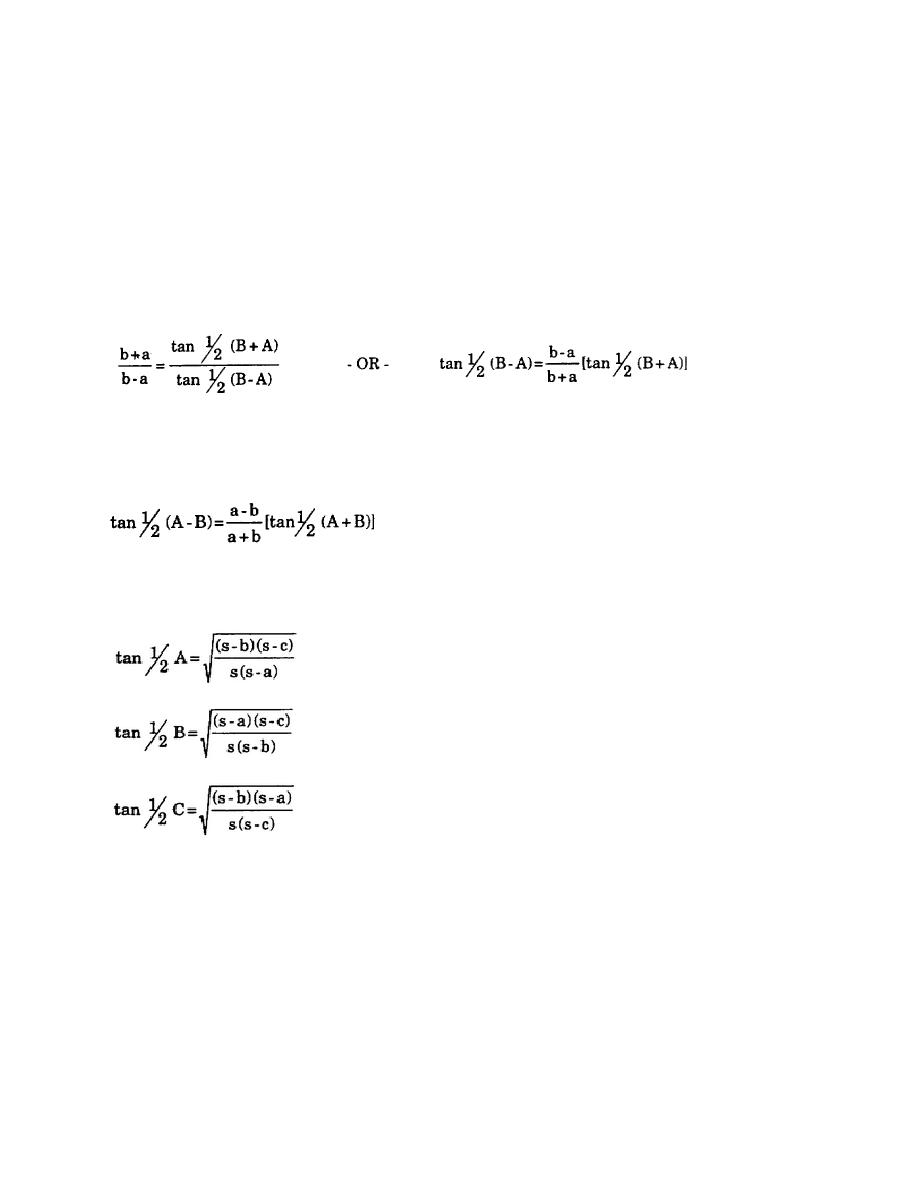
e. The tangent law states that the sum of any two sides of a triangle is to their difference as the
tangent of half the sum of their opposite angles is to the tangent of half the difference between their
opposite angles. This law is best suited for solving triangles in which two sides and the included angle
are given. The tangent law in equation form is as follows:
Since it is customary to avoid negative quantities when using the tangent law, you can rearrange the
equation to eliminate this. If in the equation above side a had been longer than side b, the equation
would have been as follows:
f. The half-tangent formulas are used to solve the angles of a triangle in cases where the given
information regarding the triangle is three sides. These formulas are as follows:
In the formulas above, s is equal to one-half the summation of the three sides (a, b, and c).
g. In the following paragraphs, there are some typical cases in which the above laws will help
you in determining the unknown quantities in oblique triangles.
4-10. Case I - One Side and Two Angles. To solve a triangle where the given information includes a
side and two angles, find the value of the third angle by subtracting the sum of the two given angles
from 180. (The sum of the interior angles of a triangle equals 180.) Then, using the sine law, solve the
two unknown sides.
4-25
EN0591


 Previous Page
Previous Page
