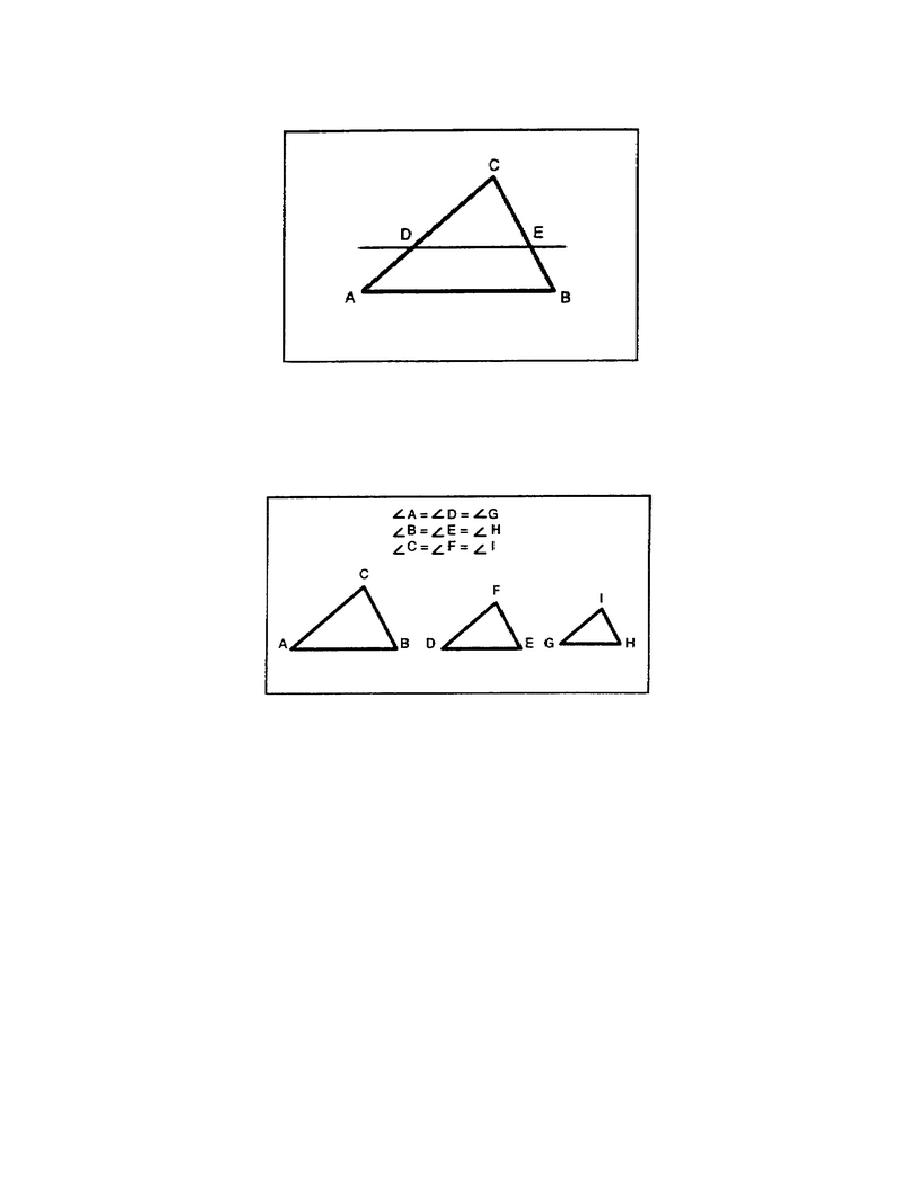
Conversely, if a line divides two sides of a triangle proportionally, it is parallel to the third side.
Figure 3-33. Line parallel to one side
b. Theorem 2. If the three angles of one triangle are equal to the three angles of another
triangle, the triangles are similar. Similar triangles are shown in Figure 3-34. Since the sum of the
interior angles of a triangle is 180€, the following corollaries can be developed from this theorem:
Figure 3-34. Equal angles
(1) Two triangles are similar if two angles of one triangle are equal to the two corresponding
angles of the other triangle.
(2) Two right angles are similar if an acute angle of one triangle is equal to an acute angle of
the other triangle.
c. Theorem 3. Two triangles are similar if their sides are respectively parallel or if their sides
are respectively perpendicular. When this condition exists, the corresponding angles of the two triangles
are similar.
d. Theorem 4. In a right triangle, if a perpendicular line is drawn from the vertex of the right
angle to the hypotenuse, the resulting two triangles are similar to the original triangle, and they are
similar to each other. This relationship is shown in Figure 3-35, page 3-20.
3-19
EN0591


 Previous Page
Previous Page
