ellipsoid can be computed. This undulation of the geoid, or distance, must be observed point by point; it
cannot be computed directly from other data.
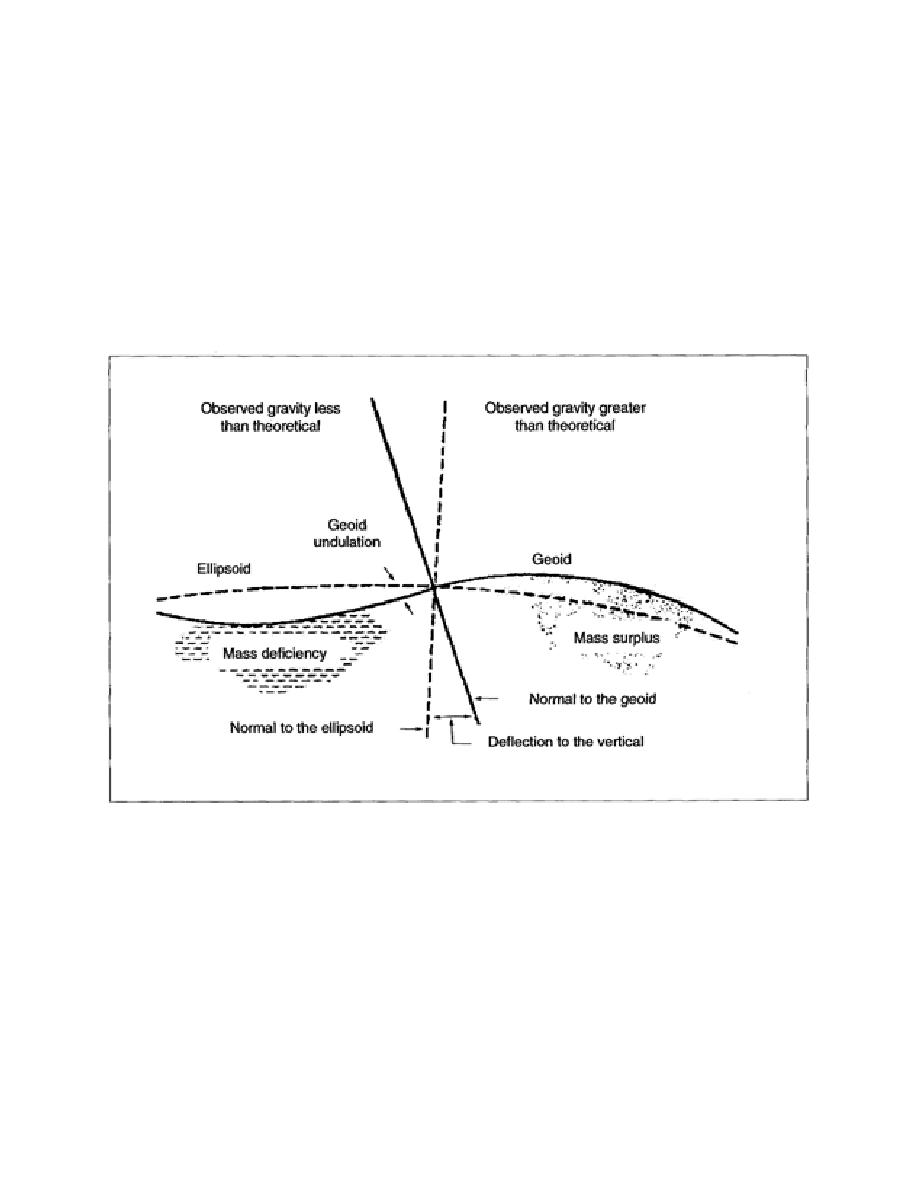
l. The difference between observed gravity and theoretical gravity at the same point is called a
gravity anomaly. Gravity anomalies can be either positive or negative. They are positive in areas of
mass surplus, where the observed gravity is greater than the theoretical value. In areas of mass
deficiency, where the observed gravity is smaller than the theoretical value, the anomaly is negative.
m. The physical basis of gravimetric studies lies in the fact that the gravity anomalies, the deflection
of the vertical, and the undulations all result from disturbing masses. The gravity anomalies can be
measured and, from these measurements, the important geodetic quantities, undulations of the geoid,
and deflections of the vertical can be computed (Figure 1-14).
Figure 1-14. Effects of Mass Anomalies on the Geoid
(1) The method for determining undulation of the geoid was developed in 1854 by a British
scientist named Sir George G. Stokes. In more recent years, a Dutch scientist named Vening Meinesz
developed the formula for computing the gravimetric deflection of the vertical (Figure 1-15, page 1-22).
1-21
EN0593


 Previous Page
Previous Page
