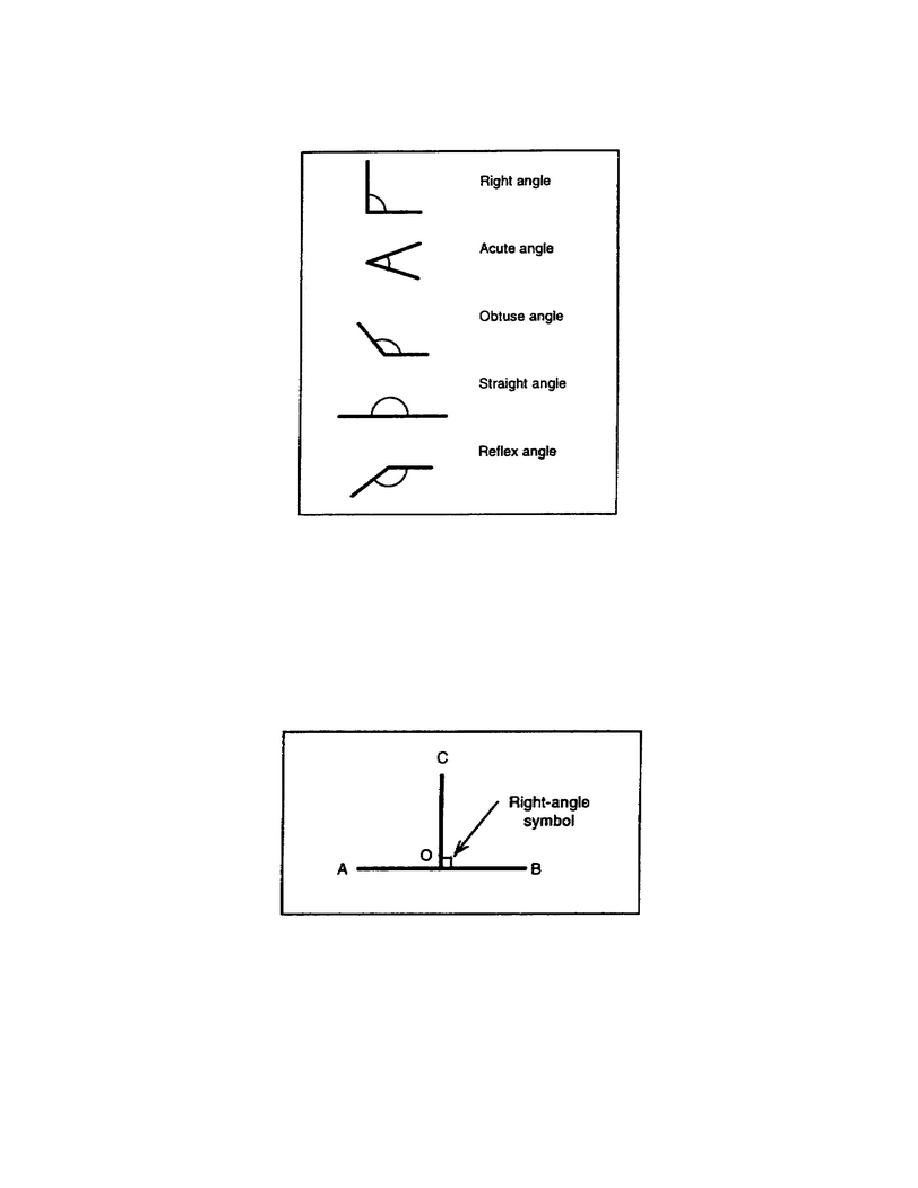
Angles can also be classified into two general groups: right and oblique. Oblique angles are all angles
other than straight and right angles.
Figure 3-3. Classes of angles
a. Adjacent Angles. Angles that have a common vertex and a common side between them are
called adjacent angles. Thus, in Figure 3-2, BOC and COD are adjacent angles; however, BOC and
AOD are not adjacent angles because they have no common side. When one straight line meets another
straight line to form two equal adjacent angles, the lines are perpendicular to each other, and the angles
are right angles. In Figure 3-4, line CO is perpendicular to line AB, and angles BOC and AOC are right
angles. The small square at the point where the lines meet is used to indicate that a right angle is formed
by the two lines.
Figure 3-4. Right angles
b. Related Angles. General relationships between angles are shown in Figure 3-5, page 3-4.
When two combined angles are equal to a right angle, they are called complementary angles. In Figure
3-5, angle AOC is a right angle, and angles AOB and BOC are complementary angles. Angle AOB is
the complement of BOC, and angle BOC is the complement of AOB. When two combined angles are
3-3
EN0591


 Previous Page
Previous Page
