d. Often, you will find that the exponent is used to show the particular root extraction of a
number that is desired. For example, the square-root extraction of a number can be indicated by the
exponent 12, the cube root by 1/3, the fourth root by 1/4, the fifth root by 15, and so forth. Examples of
the equal factor or root determination by exponents is shown below. In each example, the root 3 was
needed.
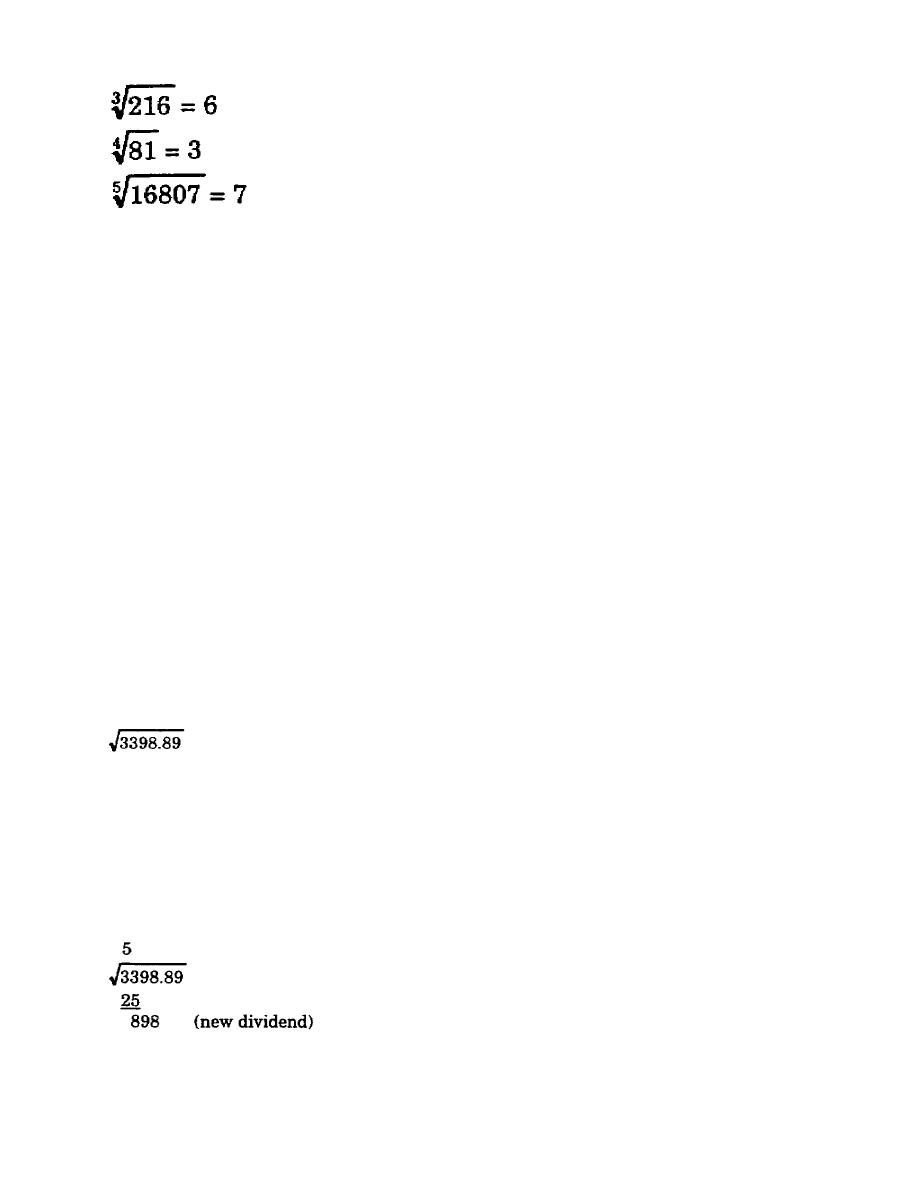
92 (9 = 3 x 3)
271/3 (27 = 3 x 3 x 3)
811/4 (81 =3 x 3 x 3 x 3)
2431/5 (243 = 3 x 3 x3 x 3 x 3)
e. According to your proficiency of common multiplication, you can determine mentally the
square root of some numbers. For example, the square root of 251/2 is 5, since 5 x 5 or 52 = 25.
Similarly, the square root of 1441/2 is 12, since 12 x 12 or 122 = 144. In other cases, however, the square
root of a number must be determined by a mathematical process. If the number is a perfect square, the
square root will be an integral number; if the number is not a perfect square, the square root will be a
continued decimal.
f. Currently, there are tables that are designed to determine the root of any number. However,
if these tables are unavailable to you while in the field, you must be able to determine the square root of
any number. For example, suppose that you have just laid out one leg of a triangle and want to calculate
the square root. The length of the leg (or side) is 3,398.89 feet. The first step would be to separate the
number (3,398.89) into 2-digit groups, starting from the decimal point and working in both directions.
g. Next, you will place the decimal point for the intended answer directly above the decimal point that
appears in the dividend under the radical sign. The square root for this number will have 1 digit for each
2-digit group. Now determine the largest number that can be squared without exceeding the first pair of
digits (33). The answer is 5, since the square of any number larger than 5 will be greater than 33. Place
the digit 5 above the first pair of digits in the dividend. Squaring 5, place the product under the first two
digits (33), and perform the indicated subtraction. Now bring down the next pair of digits as shown
below.


 Previous Page
Previous Page
